Jan 30, 2023
JEE Mains
Shift: 1
Total Questions Available: 68
Question 1
Given below are two statements : one is labelled as Assertion (A) and the other is labelled as Reason (R).
Assertion (A) : Ketoses give Seliwanoff's test faster than Aldoses.
Reason (R) : Ketoses undergo -elimination followed by formation of furfural.
In the light of the above statements, choose the correct answer from the options given below :
Options:
A)
(A) is false but (R) is trueB)
Both (A) and (R) are true but (R) is not the correct explanation of (A)C)
(A) is true but (R) is falseD)
Both (A) and (R) are true and (R) is the correct explanation of (A)Question 2
Which of the following compounds would give the following set of qualitative analysis ?
(i) Fehling's Test : Positive
(ii) Na fusion extract upon treatment with sodium nitroprusside gives a blood red colour but not prussian blue.
Options:
A)

B)

C)

D)

Question 3
Match List I with List II
| List I | List II | ||
|---|---|---|---|
| A. |  |
I. | Fittig reaction |
| B. |  |
II. | Wurtz Fittig reaction |
| C. |  |
III. | Finkelstein reaction |
| D. | IV. | Sandemyer reaction |
Choose the correct answer from the options given below :
Options:
A)
A - IV, B - II, C - III, D - IB)
A - II, B - I, C - IV, D - IIIC)
A - II, B - I, C - III, D - IVD)
A - III, B - II, C - IV, D - IQuestion 4
Match List I with List II
| List I (molecules/ions) |
List II (No. of lone pairs of e on central atom) |
||
|---|---|---|---|
| A. | I. | Three | |
| B. | II. | One | |
| C. | III. | Two | |
| D. | IV. | Zero |
Choose the correct answer from the options given below :
Options:
A)
A - II, B - I, C - IV, D - IIIB)
A - IV, B - I, C - II, D - IIIC)
A - IV, B - III, C - II, D - ID)
A - II, B - III, C - IV, D - INumerical TypeQuestion 5
Some amount of dichloromethane is added to of chloroform to prepare solution of . The concentration of is ___________ ppm (by mass).
Given :
atomic mass : C = 12
H = 1
Cl = 35.5
density of
Question 6
The major products 'A' and 'B', respectively, are

Options:
A)

B)

C)

D)

Question 7
In the wet tests for identification of various cations by precipitation, which transition element cation doesn't belong to group IV in qualitative inorganic analysis?
Options:
A)
Co2+B)
Ni2+C)
Zn2+D)
Fe3+Question 8
During the qualitative analysis of using dilute gas is evolved which turns solution (acidified with dilute ) :
Options:
A)
blueB)
blackC)
redD)
greenQuestion 9
Match List I with List II
| List I (Atomic number) |
List II (Block of periodic table) |
||
|---|---|---|---|
| A. | 37 | I. | p-block |
| B. | 78 | II. | d-block |
| C. | 52 | III. | f-block |
| D. | 65 | IV. | s-block |
Choose the correct answer from the options given below :
Options:
A)
A - II, B - IV, C - I, D - IIIB)
A - IV, B - III, C - II, D - IC)
A - IV, B - II, C - I, D - IIID)
A - I, B - III, C - IV, D - IIQuestion 10
To inhibit the growth of tumours, identify the compounds used from the following :
A. EDTA
B. Coordination Compounds of Pt
C. D - Penicillamine
D. Cis - Platin
Choose the correct answer from the option given below :
Options:
A)
A and B OnlyB)
C and D OnlyC)
B and D OnlyD)
A and C OnlyQuestion 11
What is the correct order of acidity of the protons marked in the given compounds ?

Options:
A)
B)
C)
D)
Question 12
Which of the following is correct order of ligand field strength?
Options:
A)
enB)
C)
enD)
Question 13
For molecule consider the following :
A. Number of lone pairs on oxygen is 2 .
B. FOF angle is less than .
C. Oxidation state of is .
D. Molecule is bent '' shaped.
E. Molecular geometry is linear.
correct options are:
Options:
A)
A, C, D onlyB)
A, B, D onlyC)
C, D, E onlyD)
B, E, A onlyQuestion 14
Benzyl isocyanide can be obtained by :
A. 
B. 
C. 
D. 
Choose the correct answer from the options given below :
Options:
A)
B and CB)
A and DC)
A and BD)
Only BNumerical TypeQuestion 15
The number of electrons involved in the reduction of permanganate to manganese dioxide in acidic medium is _____________.
Question 16
If the solution of the equation , is , where , are integers, then is equal to :
Options:
A)
3B)
6C)
4D)
5Question 17
Let the solution curve of the differential equation
Options:
A)
B)
C)
D)
Question 18
The minimum number of elements that must be added to the relation on the set so that it becomes symmetric and transitive is :
Options:
A)
7B)
3C)
4D)
5Question 19
Let the system of linear equations
have infinitely many solutions. Then the system
has :
Options:
A)
unique solution satisfyingB)
infinitely many solutionsC)
no solutionD)
unique solution satisfyingQuestion 20
If the coefficient of in the expansion of is equal to the coefficient of in the expansion of , where and are positive real numbers, then for each such ordered pair :
Options:
A)
a = 3bB)
ab = 1C)
ab = 3D)
a = bQuestion 21
Let a unit vector make angles with the positive directions of the co-ordinate axes , respectively, where . If is perpendicular to the plane through points and , then which one of the following is true?
Options:
A)
andB)
andC)
andD)
andQuestion 22
If an unbiased die, marked with on its faces, is thrown five times, then the probability that the product of the outcomes is positive, is :
Options:
A)
B)
C)
D)
Numerical TypeQuestion 23
Let and . Then is equal to __________.
Numerical TypeQuestion 24
The mean and variance of 7 observations are 8 and 16 respectively. If one observation 14 is omitted and a and b are respectively mean and variance of remaining 6 observation, then is equal to ___________.
Question 25
The height of liquid column raised in a capillary tube of certain radius when dipped in liquid A vertically is, . If the tube is dipped in a similar manner in another liquid of surface tension and density double the values of liquid , the height of liquid column raised in liquid would be __________ m.
Options:
A)
0.05B)
0.20C)
0.5D)
0.10Question 26
Choose the correct relationship between Poisson ratio , bulk modulus (K) and modulus of rigidity of a given solid object :
Options:
A)
B)
C)
D)
Question 27
The figure represents the momentum time () curve for a particle moving along an axis under the influence of the force. Identify the regions on the graph where the magnitude of the force is maximum and minimum respectively?
If \left(t_{3}-t_{2}\right) < t_{1}

Options:
A)
b and cB)
c and aC)
a and bD)
c and bQuestion 28
In a series LR circuit with , power factor P1. If a capacitor of capacitance C with is added to the circuit the power factor becomes P2. The ratio of P1 to P2 will be :
Options:
A)
1 :B)
1 : 3C)
1 : 2D)
1 : 1Numerical TypeQuestion 29
In an experiment for estimating the value of focal length of converging mirror, image of an object placed at from the pole of the mirror is formed at distance from the pole of the mirror. These distances are measured with a modified scale in which there are 20 small divisions in . The value of error in measurement of focal length of the mirror is . The value of is __________.
Numerical TypeQuestion 30
The general displacement of a simple harmonic oscillator is . Let T be its time period. The slope of its potential energy (U) - time (t) curve will be maximum when . The value of is ______________.
Numerical TypeQuestion 31

As per the given figure, if then the value of at this instant will be ____________ .
Question 32
If , then the value of is :
Options:
A)
B)
C)
2D)
4Numerical TypeQuestion 33
Let be the area of the larger region bounded by the curve and the lines and , which lies in the first quadrant. Then the value of is equal to ___________.
Question 34
The output waveform of the given logical circuit for the following inputs A and B as shown below, is :

Options:
A)

B)

C)

D)

Question 35
As per the given figure, a small ball P slides down the quadrant of a circle and hits the other ball Q of equal mass which is initially at rest. Neglecting the effect of friction and assume the collision to be elastic, the velocity of ball Q after collision will be :
(g = 10 m/s2)
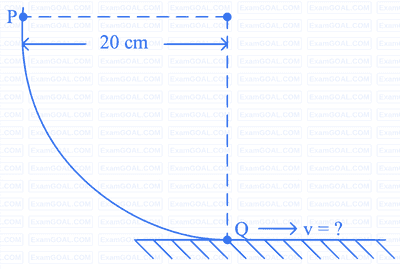
Options:
A)
0.25 m/sB)
4 m/sC)
0D)
2 m/sQuestion 36
Two isolated metallic solid spheres of radii and are charged such that both have same charge density . The spheres are then connected by a thin conducting wire. If the new charge density of the bigger sphere is . The ratio is :
Options:
A)
B)
C)
D)
Question 37
Speed of an electron in Bohr's orbit for Hydrogen atom is . The corresponding speed of the electron in orbit, in is :
Options:
A)
B)
C)
D)
Question 38
If the gravitational field in the space is given as . Taking the reference point to be at with gravitational potential . Find the gravitational potential at in SI unit (Given, that )
Options:
A)
9B)
11C)
10D)
12Question 39
A person has been using spectacles of power dioptre for distant vision and a separate reading glass of power dioptres. What is the least distance of distinct vision for this person :
Options:
A)
50 cmB)
40 cmC)
30 cmD)
10 cmNumerical TypeQuestion 40
In Young's double slit experiment, two slits and are '' distance apart and the separation from slits to screen is (as shown in figure). Now if two transparent slabs of equal thickness but refractive index and are introduced in the path of beam ) from and respectively. The central bright fringe spot will shift by ___________ number of fringes.

Numerical TypeQuestion 41
In a screw gauge, there are 100 divisions on the circular scale and the main scale moves by on a complete rotation of the circular scale. The zero of circular scale lies 6 divisions below the line of graduation when two studs are brought in contact with each other. When a wire is placed between the studs, 4 linear scale divisions are clearly visible while division the circular scale coincide with the reference line. The diameter of the wire is ______________ .
Numerical TypeQuestion 42
When 2 litre of ideal gas expands isothermally into vacuum to a total volume of 6 litre, the change in internal energy is ____________ J. (Nearest integer)
Numerical TypeQuestion 43
The energy of one mole of photons of radiation of frequency in is ___________. (Nearest integer)
[Given :
]
Question 44
If [t] denotes the greatest integer , then the value of is :
Options:
A)
B)
C)
D)
Question 45
Suppose be a differentiable function such that . If , then \sum_\limits{n=0}^{5} f(n) is equal to :
Options:
A)
6875B)
6525C)
6575D)
6825Numerical TypeQuestion 46
Let For , define . If , then is equal to ____________.
Question 47
A small object at rest, absorbs a light pulse of power and duration . Assuming speed of light as , the momentum of the object becomes equal to :
Options:
A)
B)
C)
D)
Question 48
The magnetic moments associated with two closely wound circular coils and of radius and respectively are equal if : (Where and are number of turn and current of and respectively)
Options:
A)
B)
C)
D)
Numerical TypeQuestion 49
In the following circuit, the magnitude of current I1, is ___________ A.

Numerical TypeQuestion 50
A horse rider covers half the distance with speed. The remaining part of the distance was travelled with speed for half the time and with speed for other half of the time. The mean speed of the rider averaged over the whole time of motion is . The value of is ___________.
Numerical TypeQuestion 51
If compound A reacts with B following first order kinetics with rate constant . The time taken by (in seconds) to reduce from to will be ___________. (Nearest Integer)
Numerical TypeQuestion 52
of is mixed with of . The of the mixture is ___________ . (Nearest integer)
[Given
Numerical TypeQuestion 53
Consider the cell
When the potential of the cell is at , the ratio is _____________. (Nearest integer)
Given :
Numerical TypeQuestion 54
A trisubstituted compound '', gives neutral test positive. Treatment of compound 'A' with and gives , with hydroiodic acid gives methyl iodide and with hot conc. gives a compound . Compound 'A' also decolorises alkaline . The number of bond/s present in the compound '' is _____________.
Numerical TypeQuestion 55
A solution containing of a non-volatile solute in of water boils at . The molecular mass of the solute is ___________ . (Nearest integer)
Given, water boils at for water
Numerical TypeQuestion 56
\lim_\limits{x \rightarrow 0} \frac{48}{x^{4}} \int_\limits{0}^{x} \frac{t^{3}}{t^{6}+1} \mathrm{~d} t is equal to ___________.
Numerical TypeQuestion 57
Number of 4-digit numbers (the repetition of digits is allowed) which are made using the digits 1, 2, 3 and 5, and are divisible by 15, is equal to ___________.
Numerical TypeQuestion 58
Let . Then the number of one-one functions , where denote the power set of , such that where is ____________.
Question 59
A massless square loop, of wire of resistance , supporting a mass of , hangs vertically with one of its sides in a uniform magnetic field of , directed outwards in the shaded region. A dc voltage is applied to the loop. For what value of , the magnetic force will exactly balance the weight of the supporting mass of ?
(If sides of the loop )

Options:
A)
1 VB)
VC)
10 VD)
100 VQuestion 60
The pressure and temperature ( relationship of an ideal gas obeys the equation constant. The volume expansion coefficient of the gas will be :
Options:
A)
B)
C)
D)
Question 61
The charge flowing in a conductor changes with time as . Where and are constants. Minimum value of current is :
Options:
A)
B)
C)
D)
Question 62
Heat is given to an ideal gas in an isothermal process.
A. Internal energy of the gas will decrease.
B. Internal energy of the gas will increase.
C. Internal energy of the gas will not change.
D. The gas will do positive work.
E. The gas will do negative work.
Choose the correct answer from the options given below :
Options:
A)
B and D onlyB)
C and E onlyC)
A and E onlyD)
C and D onlyQuestion 63
Electric field in a certain region is given by are :
Options:
A)
B)
C)
D)
Question 64
Match Column-I with Column-II :
| Column-I (-t graphs) |
Column-II (-t graphs) |
||
|---|---|---|---|
| A. |  |
I. |  |
| B. |  |
II. |  |
| C. |  |
III. |  |
| D. |  |
IV. |  |
Choose the correct answer from the options given below:
Options:
A)
A- II, B-III, C-IV, D-IB)
A- II, B-IV, C-III, D-IC)
A- I, B-III, C-IV, D-IID)
A- I, B-II, C-III, D-IVQuestion 65
A ball of mass rests on a vertical post of height . A bullet of mass , travelling in horizontal direction, hits the centre of the ball. After collision both travels independently. The ball hits the ground at a distance and the bullet at a distance of from the foot of the post. The value of initial velocity of the bullet will be (if ) :
Options:
A)
120 m/sB)
360 m/sC)
400 m/sD)
60 m/sNumerical TypeQuestion 66
A thin uniform rod of length , cross sectional area '' and density '' is rotated about an axis passing through the centre and perpendicular to its length with angular velocity . If value of in terms of its rotational kinetic energy is then value of is ______________.
Numerical TypeQuestion 67
A point source of light is placed at the centre of curvature of a hemispherical surface. The source emits a power of . The radius of curvature of hemisphere is and the inner surface is completely reflecting. The force on the hemisphere due to the light falling on it is ____________ .
Numerical TypeQuestion 68
A capacitor of capacitance is charged by a battery. The capacitor is disconnected from the battery and connected to another uncharged identical capacitor such that one plate of uncharged capacitor connected to positive plate and another plate of uncharged capacitor connected to negative plate of the charged capacitor. The loss of energy in this process is measured as . The value of is _____________.